Oh no. Not algebra. I can already see you click the back button but hold it there. Stay with me. Take your hand off the mouse, sit back and get ready. You’re about to find out that algebra isn’t that hard; in fact, you already know how to do it. You don’t realise it. Stick with me; we’ll get there.
Starting at the start is always the best thing to do. What is algebra? That’s simple, it is when you take a sum and replace it with letters or symbols. That is all it is. You take some of the numbers away (sometimes all) and replace them with letters or symbols.
That is what the dictionary tells us, but that’s not the whole truth. You see, algebra is used all of the time in real life. You don’t even know you’re using it sometimes. Don’t believe me? Yeah, I probably wouldn’t either, so let’s prove it.
Well, first off, each bag of crisps costs 50p, a lot, I know, but prices are getting silly these days. So if you have 3 bags of crisps and they cost 50p each, what do you do to work out the total price? You do 3 X 50p, don’t you? You know that it costs £1.50 because your head instantly works it out. So let’s try and put down what we know now.

One bag of crisps is worth 50p so as we have already said.
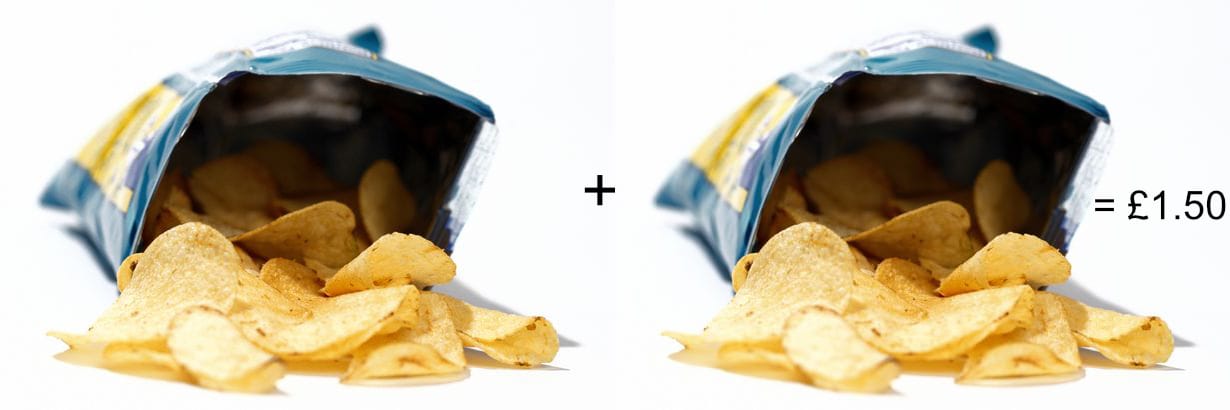
That’s pretty easy to understand, isn’t it? A picture is worth a thousand words and all that. How about if I put it across in a different way?
3C = 1.5
If I put that in front of you, could you tell me what 1c is worth? (Just a note, from here on, instead of saying 1c, or any letter for that matter, I will write c, it’s easier that way) It’s quite simple, really, isn’t it? There are 3 lots of c, which is worth 1.5, so we share 1.5 by 3. That means that c = 0.5. Or to put it as we said earlier, 3 bags of crisps cost £1.50, so 1 bag costs 50p.
Do you see how it seems a lot more complicated once letters become involved? It is just the same as with the pictures, or before that, talking about going into a shop for some crisps. Our descriptions don’t end there; let’s return to the shop.
Okay, so you remember that we went in there with £10. We already know that we’re going to be spending £1.50. So what change will we get after we have bought our crisps? £10 – £1.50 is £8.50. Not so hard, is it? What we don’t realise, though, is that when we combine everything we have done together, it is algebra.
10 – 1.5 = ?
Working out the missing number in algebra. Suppose we want to take an even bigger step.
10 – 3C = 8.5
That is the full calculation we did in our head when we bought those crisps, but the moment you see it on paper with letters, it turns into something terrifying. Let’s try and work out how to change all of that.
As we have already seen, in its most simple form, algebra is just a missing number problem. So, let’s look at a missing number problem to get ourselves in the mood. It’s always important to warm up before you start on the hard stuff; everyone knows that.
15 + ⬜ = 22
So we look at what needs to be done to find the missing number. There are several ways we could approach this, but the way I am going to do it is the way that helps us the most in the future.
Look at the sum we are being asked to do. 15 add something gives us 22. What we’re going to do to solve this is we are going to remove things until we can easily see what the empty box is worth.
How can we do that, though? Well, the easiest way to view it is to see the equals sign as a balance or a set of scales. Everything on the left-hand side of it is exactly the same as what is on the right-hand side. How does that help us to solve this sum, though? Let’s take a look.
15 + ⬜ = 22
So 15 + ⬜ – 15 = ⬜ so on the left-hand side, we are just left with ⬜ now. That means the scale is lopsided now, though. Remember, whatever we do to one side of the equals sign, we must do the same to the other.
Currently, our sum looks like this.
⬜
=
22
Notice how the 22 is significantly lower than the ⬜? That’s because we have only taken 15 from one side. Unless we take 15 from the other side, too, we will be stuck with an unbalanced equation. That doesn’t help us solve anything. We know that ⬜ doesn’t equal 22 because earlier on, 15 + ⬜ was worth 22. So what do we need to do to balance it?
That’s easy, we have to take 15 from the other side too. So if we have 22 and take 15 from it, we are left with 7. So our sum in its final state is.
⬜ = 7
Now it would have been really easy for us to realise that 7 added onto 15 makes 22, but doing it this way allows us to see what the process we are going through to get the answer. By removing the extra number from each side, we see exactly what the missing number is worth.
How is this all related to algebra, though? Algebra is all numbers and letters, isn’t it? Yeah, I suppose it is; what if we changed that sum just slightly?
15 + X = 22
It looks scary now, doesn’t it? Shall we give up? Remember what I said at the start of this, don’t click that back button. Stick with me, and we’ll get there. This is exactly the same sum as we saw before; the only difference is instead of a missing number, we have a letter. The way we would solve this is exactly the same way we solved the previous sum. We remove 15 from each side, and at the end, we are left with x = 7.
Do you see how it is exactly the same whether we have a missing number or a letter? All we need to do is ensure that whatever we do to one side of the equals sign, we do exactly the same to the other.
Let’s look at some more problems to hammer home our point. Let’s make this one a little more difficult, though.
18 – X = 7
Not too difficult, but it’s still not easy either. Remember what we did last time? We removed the same thing from both sides. So what are we going to choose to remove this time? We can get rid of the 7, the 18 or the x. It doesn’t matter which one we do, it will always give us the same final answer.
What we’re going to do, is all three. Although each choice gives us the same answer, it takes a different number of steps and has a different difficulty level.
We’ll start with the easiest choice; that makes the most sense, right? So what we’re going to remove is the 7. Remember, whatever we do to one side of the equals sign, we must do the same to the other. So if we remove the 7 from both sides, it gives us this.
11 – X = 0
(-7) (-7)
Notice the brackets in red underneath each side of the equals sign. That lets us know exactly what we have done. We removed the 7 from each side. What is remove another word for? Takeaway. So we took away 7 from each side. That leaves us with 11 – x = 0. From here, we can easily see that whatever x is worth when taken away from 11 leaves us with 0. So 11 takeaway x leaves 0. What could the x be? It’s quite obvious that x is worth 11. Meaning that our final answer is 11. We need to prove that x = 11, though. We can’t just assume.
To do that, we need to split up the 11 and -x. Or move the x over to the other side, where the 0 is. It’s the same process as removing the 7 earlier on; we can’t just make the x vanish because whatever we do to one side of the equals sign, we must do the same to the other side. So that x can never be destroyed, like energy, or wasps at a picnic, it will always be there.
How can we move it over, though? It’s a little different to removing the 7; then x isn’t a normal x. It is -x. Negative x! Let’s think about it logically, if removing the 7 (which was positive 7, even though the + sign was invisible, it was still there) involved taking away 7 from both sides, and we have -x, then we are going to have to add x to both sides. Adding an x cancels out the -x on the left-hand side and adds an x to the right-hand side. This leaves us with.
11 = X
(+X) (+X)
Which is the same as.
X = 11
There is our final answer. Simple right? What about the other choices, though? We need to check to ensure they give us the same answer. Let’s go through them quickly then, to make sure.
18 – X = 7
That is the original equation we want to work out. As always, the objective is to find out what x is worth. This time, we’re going to remove the 18.
18 – X = 7
(-18) (-18)
Taking away 18 from both sides leaves us with a different-looking equation to when we took away 7, let’s see what we’re left with.
– X = -11
Here we see a very similar-looking answer to our final answer last time. -x = -11. The thing about this is you have a negative on both sides; then you can turn them both into positives. This is because when you think about it, it makes sense. If I take away x from 0 and it gives me -11, it is obvious that x was worth 11. So if -x = -11, then it makes sense that x = 11. So our final answer is, once again.
X = 11
Okay, here we go. Time to see what answer the final choice gives us. Let’s see the original equation one last time.
18 – X = 7
This time we are going to remove the -x. Remember the first one when we were left with 11 -x = 0? We had to add an x to each side to remove the -x because the opposite of a negative is a positive. Simple.
18 = 7 + X
(+X) (+X)
Okay, so we are very close to our final answer now. We have to remove the 7 from both sides now. Remember, we aim to get the x (or whatever letter is being used) all alone.
11 = X
(-7) (-7)
That’s pretty much our final answer; we need to make it easier to read. We can do that by simply switching them around.
X = 11
There we go. Each choice had a varying number of steps to carry out, but they all came to the same answer in the end. That’s the simplest type of equation you will be asked to solve, and if you’re honest with yourself, it’s pretty easy, really, isn’t it? We’re just going to look at one a little more difficult, and then I’ll leave you alone; I promise
3X + 7 = 19
It just looks difficult, I promise. We need to use the same skills we used before. Before we do that, though, we need to recap our memories of something. Does anyone know what 3x means? 3x means 3 X x. 3 multiplied by x if seeing all the different sizes of x on the screen makes things difficult to read. 3x means 3 times x. Now we know that we can start solving this equation.
We’re not going to go with different choices this time. While we could reverse the multiplication first by dividing, it just makes things a massive pain in the neck. Whenever we see an equation like this, we ALWAYS ensure that the x value (in this case, 3x) is alone first. So in this particular example, we will remove 7 from both sides.
3X = 12
(-7) (-7)
Do you see how much neater that would be if we divided both sides by 3 first? If we had done that first, we would have been working with fractions or decimals. This time we have two nice and clean sides to work with. Simple.
So our next step is removing that annoying 3 from in front of the x. Once we’ve done that, we’re all finished. Remember what 3x meant? That’s right, 3x means 3 times x. So to remove those times, we will do the opposite (to both sides, remember) and divide. So that gives us a final answer.
X = 4
(÷3) (÷3)
There we have it. We’re all finished. It wasn’t that painful, was it? I might have lied a little; we’re not totally finished; let’s go over the important things to remember. First off, don’t panic. Algebra isn’t going to kill you; it isn’t going to trick you; as long as you remember just to use what you’ve learned, you will be fine. Secondly, remember that when it all boils down to it, it’s just a missing number problem. You’re not finding x; you’re finding a missing number named x. That’s it. You know how to work out missing numbers; you do it daily when you go to the shop. Finally, and this is the most important part, remember that whatever you do to one side of the equation, you must do exactly the same to the other. As long as you remember all of this, you will be completely fine.











